_________________________________________________________________________________
Calculus  II                                                          Test #1                                                  March 3, 2004

Name____________________                     R.  Hammack                                                Score ______
_________________________________________________________________________________
(1) Find the following antiderivatives.

(a) ∫ (1 + x^3) dx = x + x^4/4 + C

(b) ∫ (cos(x) + 3sec^2(x)) dx = sin(x) + 3tan(x) + C

(c) ∫ (e^x + π) dx = e^x + π x + C

(d) ∫x/(x^4 + 1) dx = 1/2∫ (2x)/((x^2)^2 + 1) dx = 1/2∫1/(u^2 + 1) du = 1/2tan^(-1)(u) + C = 1/2tan^(-1)(x^2) + C

u = x^2  du = 2 x

(e) ∫ (x^4 + 1)/xdx = ∫ (x^4 /x + ( 1)/x) dx = ∫ (x^3 + ( 1)/x) dx = x^4/4 + ln | x | +C

(f) ∫e^(x + 1)^(1/2)/(x + 1)^(1/2) dx = 2∫e^(x + 1)^(1/2) 1/(2 (x + 1)^(1/2)) dx = 2∫e^udu = 2e^u + C = 2e^(x + 1)^(1/2) + C

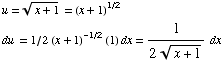
u = (x + 1)^(1/2) = (x + 1)^(1/2)  du = 1/2 (x + 1)^(-1/2) (1) dx = 1/(2 (x + 1)^(1/2)) dx


(2) Use Part 2 of the Fundamental Theorem of Calculus to write an antiderivative of f(x) = ln(cos(x) + 2)

F(x) = ∫_1^x ln(cos(t) + 2) dt


(3) Find the following definite integrals. (You may use any technique. Sometimes area may be the best approach.)

(a)   ∫__ (-1)^1 (x + 1) dx =[ x^2/2 + x] _ (-1)^1 =[ 1^2/2 + 1] -[ (-1)^2/2 - 1] = 2


(b)    ∫__1^31/x^2dx =[ -1/x] _1^3 = -1/3 - -1/1 = 2/3


(c)  ∫_0^(1/2) 1/(1 - x^2)^(1/2) dx =[ sin^(-1)(x)] _0^(1/2) = sin^(-1)(1/2) - sin^(-1)(0) = π/6 - 0 = π/6


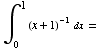
(d)  ∫__0^1 (x + 1)^(-1) dx =[ ln | x + 1 |   ] _0^1 = ln(2) - ln(1) = ln(2)


(e)  ∫_ (-2)^( 2) (4 - x^2)^(1/2) dx =(π 2^2)/2 = 2π
(Because graph of integtrand is half a circle of radius 2)

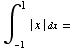
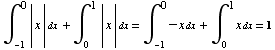
(f)  ∫__ (-1)^1 | x | dx = ∫__ (-1)^0 | x | dx + ∫__0^1 | x | dx = ∫__ (-1)^0 -x dx + ∫__0^1x dx = 1


(4)  Use the definition of the definite integral to write  ∫_1^( 2) (ln(x) + x )^(1/2) dx   as a limit of Riemann sums.
(You do not need to find the value of this integral -- just write down the limit,)   

∫_1^( 2) (ln(x) + x )^(1/2) dx = Underscript[lim , n∞] Underoverscript[∑ , k = 1, arg3] (ln(x_k^*) + x_k^* )^(1/2) Δx

By setting Δx = (2 - 1)/n = 1/n, and x_k^* = 1 + k/n, this becomes

∫_1^( 2) (ln(x) + x )^(1/2) dx = Underscript[lim , n∞] Underoverscript[∑ , k = 1, arg3] (ln(1 + k/n) + 1 + k/n )^(1/2) k/n
(5)  Find the average value of f(x) = x^(1/2) on the interval [0, 4].


1/(4 - 0) ∫__0^4x^(1/2) dx = 1/4∫__0^4x^(1/2) dx = 1/4[x^(3/2)/(3/2)] __0^4 = 1/4[(2x^(1/2)^3)/3] __0^4 = 1/4[(24^(1/2)^3)/3 - (20^(1/2)^3)/3] = 4/3



(6) Find the derivatives of the following functions.

(a)   F(x) =    ∫__2^xtan (t^(1/2)) dt      F ' (x) = tan (x^(1/2))


(b)   G(x) =    ∫__2^ln(x) tan(t^(1/2)) dt    G ' (x) = tan(ln(x)^(1/2)) 1/x


(7) Suppose a particle moves along the s-axis in such a way that its acceleration at time t seconds is a(t) = 4cos(t)units per second per second. Suppose that  v(0) = -1 and  s(0) = 3.  Find the position function s(t).


v(t) = ∫a(t) dt = ∫4cos(t) dt = 4sin(t) + C
Now, -1 = v(0) = 4sin(0) + C, which becomes -1 = C, so the velocity function is v(t) = 4sin(t) - 1.

Next, s(t) = ∫v(t) dt = ∫ (4sin(t) - 1) dt = -4cos(t) - t + C.
Now, 3 = s(0) = -4cos(0) - 0 + C, which becomes 3 = -4 + C, and C=7.

Therefore, the position function is s(t) = -4cos(t) - t + 7.

(8) Suppose that    ∫_3^2f(x) dx = 5  and  ∫_2^6f(x) dx = 7.

(a)    ∫_2^32f(x) dx = 2∫_2^3f(x) dx = -2∫_3^2f(x) dx = -10


(a)    ∫_3^6f(x) dx =

Note that ∫_2^6f(x) dx = ∫_2^3f(x) dx + ∫_3^6f(x) dx
Thus,      ∫_2^6f(x) dx = -∫_3^2f(x) dx + ∫_3^6f(x) dx
Plugging in the known information, we get
7 = -5 + ∫_3^6f(x) dx, so ∫_3^6f(x) dx = 12