_______________________________________________________________________
Calculus I                                                   Diagnostic Quiz                      February 9, 2004

Name____________________                   R.  Hammack                              Score ______
_______________________________________________________________________

(1) Find all solutions:     x^2 - 1 = 3
   x^2 - 4 = 0  (x - 2) (x + 2) = 0
   Solutions: x = 2 and x = -2


(2) Find all solutions:    x^2 - x - 1 = 0
It's a quadratic set equal to 0 and it doesn't factor,
so we use the quadratic formula:
Solutions: x = (-b ± (b^2 - 4a c)^(1/2))/(2a) = (1 ± ((-1)^2 - 4 (1) (-1))^(1/2))/(2 (1)) = (1 ± (1 + 4)^(1/2))/2 = (1 ± 5^(1/2))/2

Thus, there are two solutions: x = (1 + 5^(1/2))/2and  x = (1 - 5^(1/2))/2.


(3) Find all solutions:      x^3 + 5x^2 + 7x = x
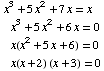
   x^3 + 5x^2 + 7x = xx^3 + 5x^2 + 6x = 0x(x^2 + 5x + 6) = 0x(x + 2) (x + 3) = 0
  
  Solutions: x=0, x= -2 and x= -3


(4)   cos(-π/6) = 3^(1/2)/2


(5)   Simplify as much as possible:  (2x - (x - 1))/(2x(x + 1)) =(2x - x + 1)/(2x(x + 1)) = (x + 1)/(2x(x + 1)) = 1/(2x)


(6) A straight line has slope 2 and x-intercept 3. What is its y-intercept?
Call the y-intercept b.
The equation of the line is y=2x+b.
The x-intercept being 3 means y = 0 when x=3.
Plug this in and the equation becomes: 0=2(3)+b, or 0=6+b.
From this, the y-intercept is b = -6.