Calculus I Test #3 December 1, 2004
Name____________________ R. Hammack Score ______
_____________________________________________________________________________________
(1) Find the inverse of the function

(2) Is the function
No. It's not one-to-one:
so g(1) = g(-1).
It fails the H.L.T.
(3) Find the equation of the tangent line to the graph of
Slope at x is y ' =
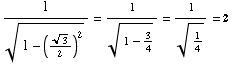
Point of tangency is
Point-Slope formula:
ANSWER:
(4) Solve the equation
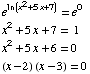
SOLUTIONS: 2, 3
(5) Simplify each expression as much as possible.
(a)
(b)
(c)
(d)
(e)
(6) The graph of the derivative of a function f is given.
![[Graphics:HTMLFiles/T3F04D_24.gif]](HTMLFiles/T3F04D_24.gif)
In each case, indicate whether the ? should be replaced with the symbol
(a) f(1) ? f(3) ANSWER: > , because f decreases between 1 and 3 (its derivative is negative there).
(b) f '(1) ? f '(3) ANSWER: =, by reading straight from the graph.
(c) f "(1) ? f "(3) ANSWER: <, by looking at slope on the graph of f '
(7) Find the derivatives.
(a)
(b)
(c)
(d)
(e)
(f)
(g)

(h)
![y = x^x ln(y) = ln(x^x) ln(y) = x ln(x) d/dx[ln(y)] = d/dx[x ln(x)] 1/yy ' = ln(x) + x1/x y ' = y (ln(x) + 1) y ' = x^x (ln(x) + 1)](HTMLFiles/T3F04D_43.gif)
(8) Consider the function
(a) List all critical points of f.
From this you can read off the critical points as 0 and -3
(b) Find the intervals on which f increases/decreases.
-3 0
---|------|-----
- - - - - - + + +f '(x)
f increases between 0 and infinity.
f decreases between negative infinity and 0
(c) Find the intervals on which f is concave up/down.
-3 -1
---|------|-----
++ - - - + + +f ''(x)
f is concave down on [-3,-1]
Elsewhere, f is concave up
(d) Locate and identify all extrema of f .
By first derivative test (see part b above) there is a relative minimum at x = 0.
There is no relative maximum.
(e) List the locations (x-values) of all inflection points of f.
By part c above, the locations are -3 and -1.