Calculus I Test #3 December 1, 2004
Name____________________ R. Hammack Score ______
_____________________________________________________________________________________
(1) Find the inverse of the function
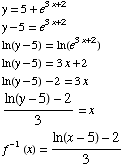
(2) Is the function
Since
Hence it passes the Horizontal Line Test, so it's invertible.
(3) Find the equation of the tangent line to the graph of
Slope at x is y ' =
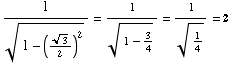
Point of tangency is
Point-Slope formula:
ANSWER:
(4) Solve the equation
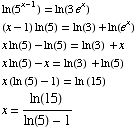
(5) Simplify each expression as much as possible.
(a)
(b)
(c)
(d)
(e)
(6) The graph of the derivative of a function f is given.
![[Graphics:HTMLFiles/index_23.gif]](HTMLFiles/index_23.gif)
In each case, indicate whether the ? should be replaced with the symbol
(a) f(1) ? f(3) ANSWER: > , because f decreases between 1 and 3 (its derivative is negative there).
(b) f '(1) ? f '(3) ANSWER: =, by reading straight from the graph.
(c) f "(1) ? f "(3) ANSWER: <, by looking at slope on the graph of f '
(7) Find the derivatives.
(a)
(b)
(c)
(d)
(e)
(f)
(g)
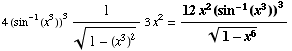
(h)
![y = x^x ln(y) = ln(x^x) ln(y) = x ln(x) d/dx[ln(y)] = d/dx[x ln(x)] 1/yy ' = ln(x) + x1/x y ' = y (ln(x) + 1) y ' = x^x (ln(x) + 1)](HTMLFiles/index_42.gif)
(8) Consider the function
(a) List all critical points of f.
From this you can read off the critical points as 0 and -3
(b) Find the intervals on which f increases/decreases.
-3 0
---|------|-----
- - - - - - + + +f '(x)
f increases between 0 and infinity.
f decreases between negative infinity and 0
(c) Find the intervals on which f is concave up/down.
-3 -1
---|------|-----
++ - - - + + +f ''(x)
f is concave down on [-3,-1]
Elsewhere, f is concave up
(d) Locate and identify all extrema of f .
By first derivative test (see part b above) there is a relative minimum at x = 0.
There is no relative maximum.
(e) List the locations (x-values) of all inflection points of f.
By part c above, the locations are -3 and -1.